Standards for Mathematical Practice » Attend to precision. Mathematically proficient students try to communicate precisely to others. They try to use clear definitions in discussion with others and in their own reasoning. They state the meaning of the symbols they choose, including using the equal sign consistently and appropriately. They are careful about specifying units of measure, and labeling axes to clarify the correspondence with quantities in a problem. They calculate accurately and efficiently, express numerical answers with a degree of precision appropriate for the problem context.

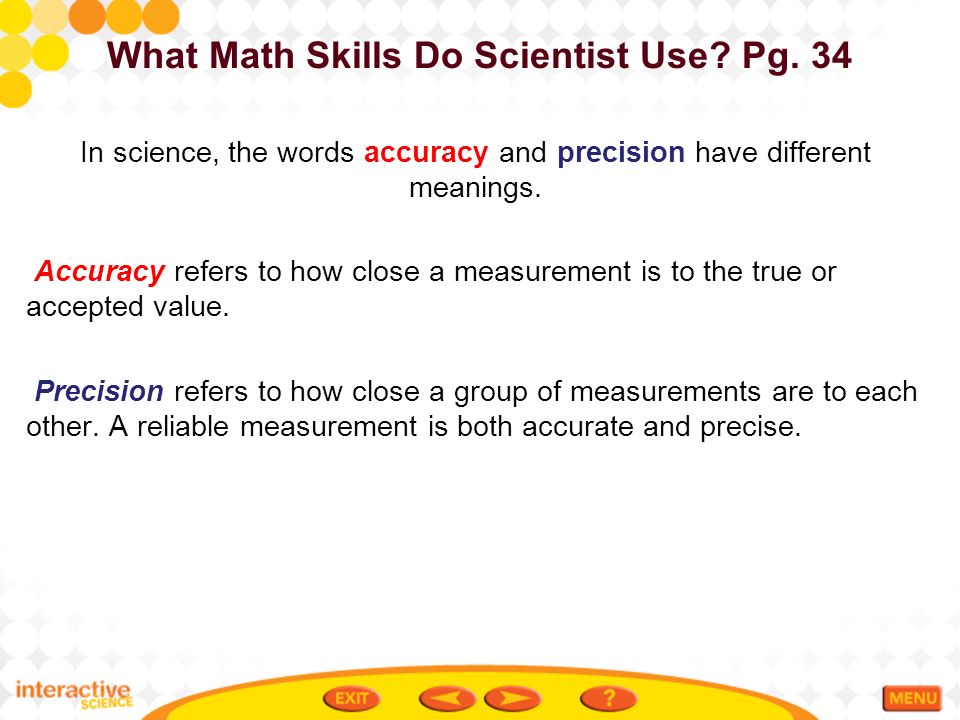
Standards for Mathematical Practice » Attend to precision. Print this page. Mathematically proficient students try to communicate precisely to others. Accuracy is how close a measured value is to the actual (true) value. Precision is how close the measured values are to each other. So, if you are playing soccer and you always hit the left goal post instead of scoring, then you are not accurate, but you are precise! ACcurate is Correct (a bullseye).

In the elementary grades, students give carefully formulated explanations to each other. By the time they reach high school they have learned to examine claims and make explicit use of definitions.

Precision refers to how much information is conveyed by a number (in terms of number of digits) whereas accuracy is a measure of 'correctness'. Let's take the π approximation 22/ 7, for our purposes, 3.142857143. For your specific questions: • a number that is accurate but not precise: 3.14.

That's certainly accurate in terms of closeness, given the precision available. There is no other number with three significant digits that is closer to the target (both 3.13 and 3.15 are further away from the real value). • a number that is precise but not accurate: 567890. That's much more precise since it conveys more information. Unfortunately its accuracy is way off since it's nowhere near the target value. • a number that is both accurate and precise: 3.142857143. You can get more precise (by tacking zeros on the end) but no more accurate.

Of course, that's if the target number is actually 3.142857143. If it's 22/ 7, then you can get more accurate and precise, since 3.142857143 * 7 = 01. The actual decimal number for that fraction is an infinitely repeating one (in base 10): 3. 157 157 142857. Program Mentor Mentee Kementerian Kesihatan Malaysia. And so on, so you can keep adding precision and accuracy in that representation by continuing to repeat that group of six digits. Or, you can maximise both by just using 22/ 7.